[BA 4th Sem Question Papers, Dibrugarh University, 2014, Mathematics, Major, A: Linear Programming, B: Analysis - II Multiple Intergral]
A: LINEAR PROGRAMMING
(Marks: 45)
1. (a) Is the solution 1
a basic solution of the equations
(b) Show that a hyperplane is a convex set.
(c) A factory is engaged in manufacturing two products – A and B which involve lathe work, grinding and assembling. The cutting, grinding and assembling times required for one unit of A and 2, 1 and 1 hours respectively and for one unit of B are 3, 1 and 3 hours respectively. The profits on each unit of A and B are Rs. 2 and Rs. 3 respectively. Assuming that 300 hours of lathe time, 300 hours of grinding time and 240 hours of assembling time are available, formulate a linear programming problem in terms of maximizing the profit on the items manufactured.
(d) Use the graphical method to solve the following LP problem:
Minimize
Subject to the constraints
2. (a) Write ‘true’ or ‘false’: “If a linear programming problem has a feasible solution, then it has a basic feasible solution.”
(b) Express the following LPP in the standard form by adding additional variables to the left side of each constraint and assign a zero-cost coefficient to these in the objective function:
Maximize
2
2 Subject to the constraints
(c) Solve by simplex method: 5
Maximize
Subject to the constraints
(d) Solve by two-phase method: 7
Minimize
Subject to the constraints
Or
Solve by Big-M method:
Minimize
Subject to the constraints
3. (a) Fill in the blank: If any of constraints in the primal problem is a perfect equality, then the corresponding dual variable is ____. 1
(b) Write ‘true’ or ‘false’: “An LPP has a finite optimal solution if and only if there exist feasible solutions to both the primal and dual problems.”
(c) Find the dual of the following primal problem:
Minimize
Subject to the constraints
(d) Write down the correspondence rules between the primal problem and the dual problem.
Or
If the dual problem has no feasible solution and the primal problem has a feasible solution, then prove that the primal objective function is unbounded.
4. (a) What do you mean by rim condition of a transportation problem? 1
(b) Examine with diagram whether the following ordered set of cells forms a loop or not: 2
(c) Obtain an optimal solution to the following transportation problem by the MODI method: 9
 |  |  | Supply | |
 | 16 | 20 | 12 | 200 |
 | 14 | 8 | 18 | 160 |
 | 26 | 24 | 16 | 90 |
Demand | 180 | 120 | 150 | 450 |
Or
Write short notes on: 4 ½ x2=9
- Least cost method.
- Vogel’s approximation method.
B: ANALYSIS – II
(Multiple Integral)
(Marks: 35)
5. (a) Write ‘true’ or ‘false’: “A periodic function of bounded variation can be expressed as a Fourier series.” 1
(b) Find the Fourier coefficient 
(for 
odd) of the following function with period 
2
(for odd) of the following function with period 2
(c) Find the Fourier series generated by the periodic function 
of period
. 3
of period. 3 (d) Find the trigonometrically series which converges in 
to the function 4
to the function 4
Or
Expand the function 
in a Fourier series in 
in a Fourier series in 6. (a) Define line integral in two-dimensional space. 1
(b) Evaluate the integral 
taken along the line segment from 
to 
. 2
taken along the line segment from to . 2 (c) Prove that a bounded function 
on a region
, having an infinite number of discontinuities lying on a finite number of smooth curves, is integrable on 
5
on a region, having an infinite number of discontinuities lying on a finite number of smooth curves, is integrable on 5Or
Evaluate 
over the part of the plane bounded by the lines 
and the parabola
.
over the part of the plane bounded by the lines and the parabola. (d) With the help of Green’s theorem, prove that the line integral
taken in the positive direction over any closed contour with the origin inside it, is equal to 
. 5
. 5Or
Compute the double integrals of the function 
over 
over 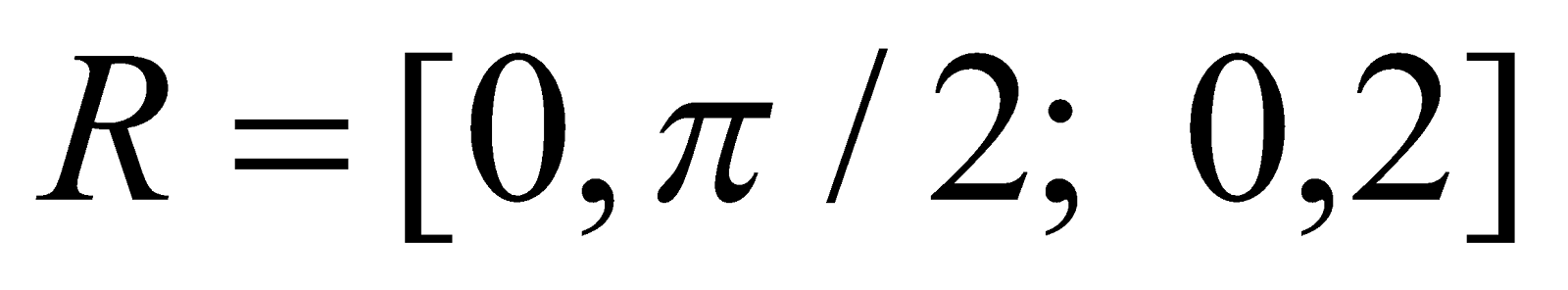7. (a) State Stokes’ theorem. 1
(b) Find the length of the curve
,
, 
,
2
,, , 2 (c) Compute the surface area of the sphere 
4
4Or
Show that
is equal to 3/8, where S is the outer surface of the sphere 
in the 1st octant.
in the 1st octant. (d) State and prove Gauss’ theorem. 5
Or
Also Read: Dibrugarh University Question Papers
Evaluate the surface integral by Gauss’ theorem of the following:
Over the sphere 
***
Post a Comment
Kindly give your valuable feedback to improve this website.