2014
(November)
MATHEMATICS
(General)
Course: 501
[(A) Analysis – II, (B) Mechanics]
Full Marks: 80
Pass Marks: 32
Time: 3 hours
The figures in the margin indicate full marks for the questions
1. (a) Write the necessary conditions for a function 
to be analytic. 1
to be analytic. 1
(b) Show that the function 
is not analytic. 3
is not analytic. 3
(c) Derive the polar form of Cauchy-Riemann equations of an analytic function.
Or
If 
, then find 
such that 
is analytic.
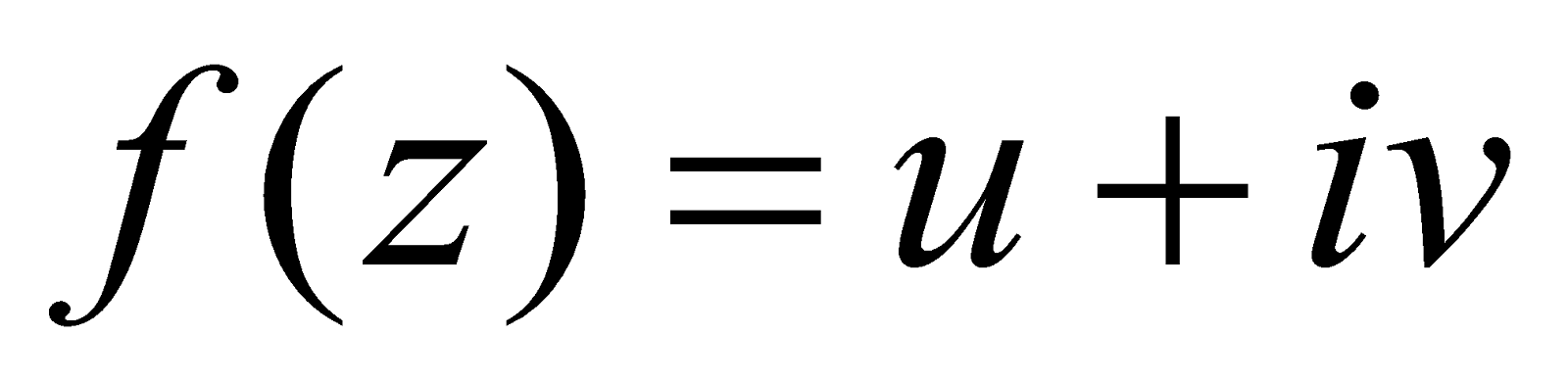, then find such that is analytic.
2. (a) Define Jordan curve.
(b) Evaluate 
from 
to 
along the curve
.
from to along the curve.
(c) State and prove Cauchy’s theorem.
Or
If 
is analytic inside and on the boundary C of a simply-connected region R, then prove that
is analytic inside and on the boundary C of a simply-connected region R, then prove that 
3. (a) State Taylor’s theorem. 1
(b) Define pole of an analytic function. 1
(c) Define residue of an analytic function. 1
(d) Find the residue of 
at the point
. 2
at the point. 2
(e) Find the poles of the function
in a unit circle. 2
(f) Expand 
in a Laurent’s series for
.
in a Laurent’s series for.
Or
Evaluate
, 
using contour integration.
, using contour integration.
(B) Mechanics
(Marks: 45)
(a) Statics
4. (a) Define the central axis of a system of coplanar forces.
(b) Define pitch.
(c) Write the resultant of wrench of two given forces 
and 
inclined at an angle 
. 2
and inclined at an angle . 2
(d) Find the equation of the central axis of a system of forces acting on a rigid body. 6
Or
Prove that any system of forces acting on a rigid body can be reduced to a single force together with a couple whose axis is along the direction of the single force.
5. (a) Define virtual work. 1
(b) Define axis of catenary. 1
(c) Find the relation between 
and 
in a common catenary.
and in a common catenary.
(d) Derive intrinsic equation of a common catenary.
Or
Find the work done by tension of a light rod.
(b) Dynamics
6. (a) Define frequency of a simple harmonic motion.
(b) Find the radial velocity and transverse velocity of a particle moving in a plane curve at any point
.
.
Or
A point moves in a plane curve, so that its tangential and normal accelerations are equal. The angular velocity of the tangent is constant. Find the curve.
7. (a) Define central force. 1
(b) Find the law of force to the pole if the path of the particle is 
. 6
. 6
Or
A particle falls under gravity from rest in a medium whose resistance varies as the velocity. Find a relation between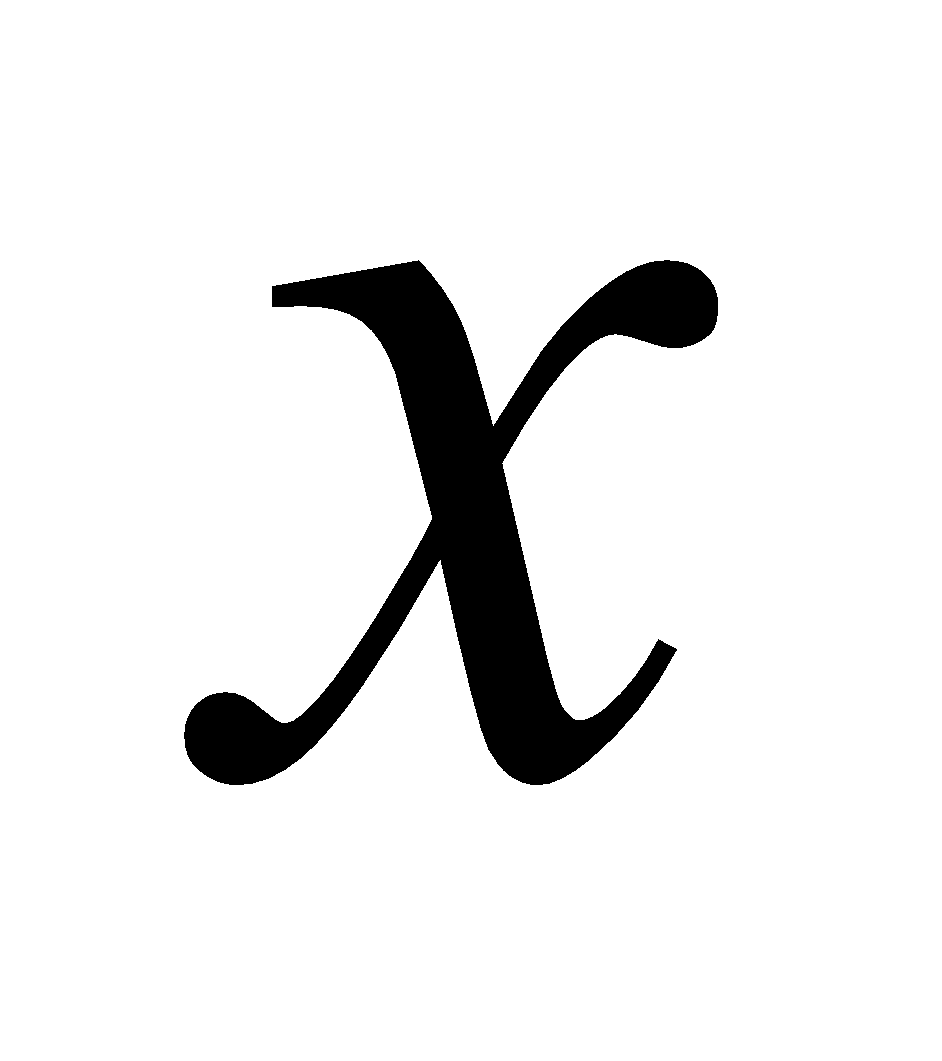 
and
.
and.
8. (a) Define moment of inertia of a body about a line. 2
(b) Write the product of inertia of a body of mass 
with respect to 
and 
axes.
with respect to and axes.
(c) Find the moment of inertia of a rectangular lamina about 
, which passes through the centre of the lamina; 
being parallel to one of its edges.
, which passes through the centre of the lamina; being parallel to one of its edges.
***
Post a Comment
Kindly give your valuable feedback to improve this website.